本記事では、以下の三角関数の極限について導出過程を示します。
対象読者:高校生
必要な知識:高校数学 三角関数、極限
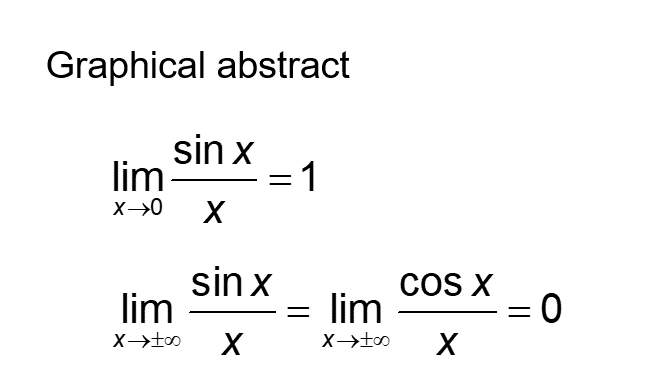
sin関数の極限
sin関数の極限として、以下の式が成立します。
本ページではこの式の導出過程を示します。
$$ \lim _{x \to 0} \frac{\sin x}{x}=1 \tag{1}$$
$$ \lim _{x \to \pm \infty} \frac{\sin x}{x} = \lim _{x \to \pm \infty} \frac{\cos x}{x}=0 \tag{2} $$
Eq. (1)の導出(\(x \to 0\)の極限の導出)
Eq. (1)の導出にあたり、まずは\( 0<x<\pi/2\)を考えて、下図の二つの三角形と単位円上の扇を考えます。
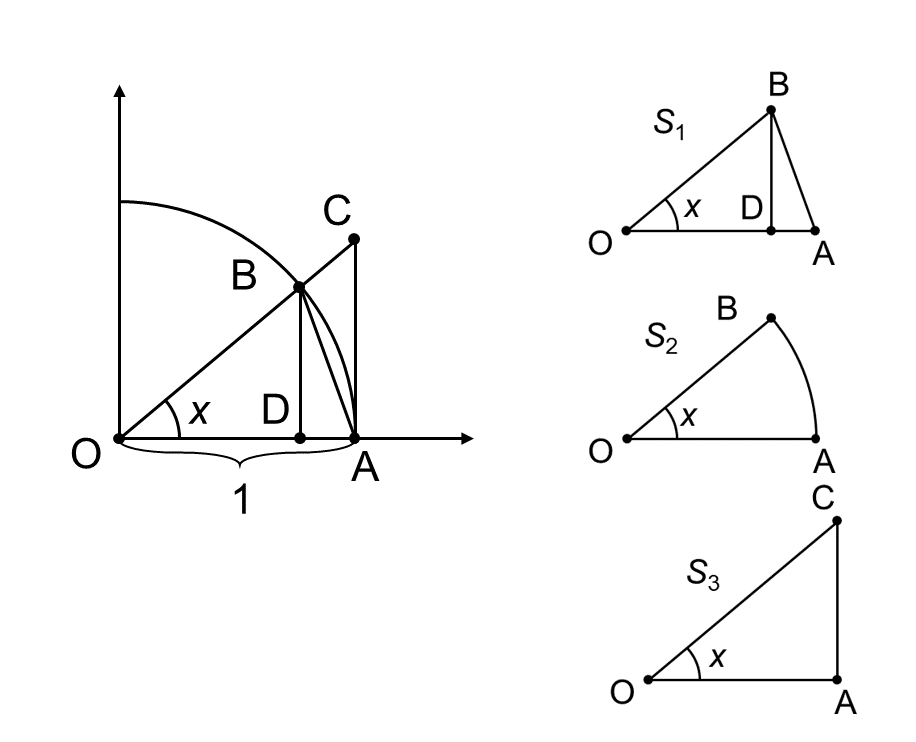
△OAB, 扇OAB, △OACの面積をそれぞれ\(S_1, S_2, S_3\)とすると、上図より以下の大小関係が成立します。
$$ S_1<S_2<S_3 \tag{1-1} $$
それぞれの面積は以下の式で与えられます。
$$
\begin{eqnarray}
S_1
&=& \frac{1}{2} \cdot OA \cdot BD \\
&=& \frac{1}{2} \cdot 1 \cdot 1 \sin x \\
&=& \frac{1}{2} \sin x
\end{eqnarray}
$$
$$
\begin{eqnarray}
S_2
&=& \pi \cdot 1^2 \cdot \frac{x}{2\pi} \\
&=& \frac{1}{2}x \\
\end{eqnarray}
$$
$$
\begin{eqnarray}
S_3
&=& \frac{1}{2} \cdot OA \cdot CA \\
&=& \frac{1}{2} \cdot 1 \cdot 1 \tan x \\
&=& \frac{1}{2} \tan x
\end{eqnarray}
$$
Eq. (1-1)にこれらを代入すると以下のようになります。
$$ S_1<S_2<S_3 $$
$$ \frac{1}{2} \sin x<\frac{1}{2}x<\frac{1}{2} \tan x $$
$$ \sin x<x<\frac{\sin x}{\cos x} $$
$$ \frac{\sin x}{x}<1<\frac{1}{\cos x}\frac{\sin x}{x} $$
この式を整理すると以下の式が得られます。
$$ \cos x<\frac{\sin x}{x}<1 \tag{1-2} $$
\(x \to +0\)の極限を考えます。
$$ \lim_{x \to +0} \cos x=\lim_{x \to +0} 1=1 $$
Eq. (1-2)に対する、はさみうちの原理より以下の極限が得られます。
$$ \lim_{x \to +0} \frac{\sin x}{x}=1 $$
続いて、\(x \to -0\)の極限を考えるため、\(y=-x\)を考えます。
$$ \lim_{x \to -0} \frac{\sin x}{x} = \lim_{y \to +0} \frac{\sin (-y)}{-y} $$
三角関数の関係式とEq. (1-2)を用いて変形します。
三角関数の関係式
$$ \sin \left( -x \right) = – \sin x \tag{1-3} $$
$$
\begin{eqnarray}
\lim_{x \to -0} \frac{\sin x}{x}
&=& \lim_{y \to +0} \frac{\sin (-y)}{-y} \\
&=& \lim_{y \to +0} \frac{-\sin y}{-y} \\
&=& \lim_{y \to +0} \frac{\sin y}{y} \\
&=& 1
\end{eqnarray}
$$
\(x \to \pm 0\)の極限より、Eq. (1)が導かれます。
$$ \lim _{x \to 0} \frac{\sin x}{x}=1 \tag{1} $$
Eq. (2)の導出(\(x \to \pm \infty\)の極限の導出)
三角関数の値域より、以下の関係式が成立します。
三角関数の値域
$$ -1 \leq \sin x \leq 1 \tag{2-1} $$
$$ -1 \leq \cos x \leq 1 \tag{2-2} $$
$$ -\frac{1}{x} \leq \frac{\sin x}{x} \leq \frac{1}{x} $$
$$ -\frac{1}{x} \leq \frac{\cos x}{x} \leq \frac{1}{x} $$
以下の極限から、はさみうちの原理よりEq. (2)が導かれます。
極限
$$ \lim_{x \to \pm \infty} -\frac{1}{x}=\lim_{x \to \pm \infty} \frac{1}{x}=0 \tag{2-3} $$
$$ \lim _{x \to \pm \infty} \frac{\sin x}{x} = \lim _{x \to \pm \infty} \frac{\cos x}{x}=0 \tag{2} $$
関連ページ
高校生向け数学記事の一覧